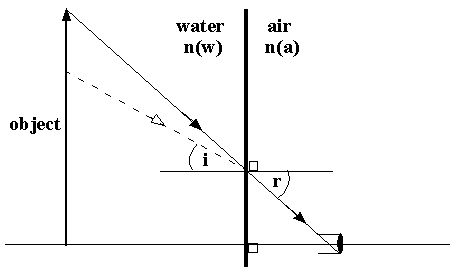
The angular field of view of a lens facing a planar air/water interface is less than its field of view in air. In the figure below, an object is shown which exactly fills the lens' in-air half-angle of view 'r'. The limiting light ray has been drawn from the top of the object to the lens. When the same object is immersed in water, rays from the top of the object can no longer reach the lens, because they are refracted away.
sin(i) = [n(a)/n(w)]*sin(r)

in-air angular field of view: 180 140 100 60 30 in-water angular field of view: 97 90 70 44 22
For example, the underwater field of view of a 180-degree 'fisheye' lens is only 97 degrees behind a plane port.